| |
When a guitar string is
plucked, one observes that the string vibrates according to the patterns
shown in the animation below. When the string is pulled to one side by the
finger, the displacement of the string moves off as a travelling wave in
both directions. After having reached the bridge at either end of the
string, the waves are reflected back. These waves travel back and forth
along the string but the resultant motion is a standing wave due to the
addition of the left and right travelling components.
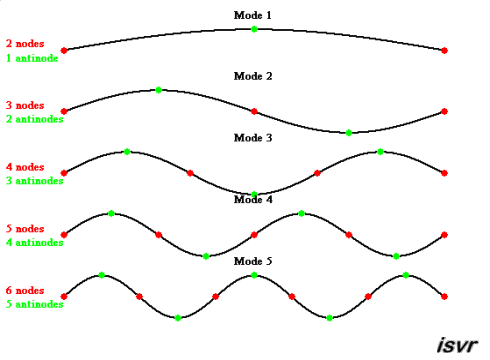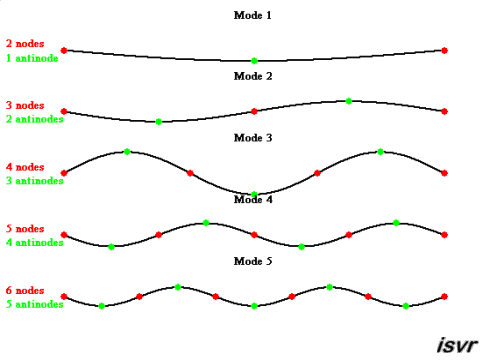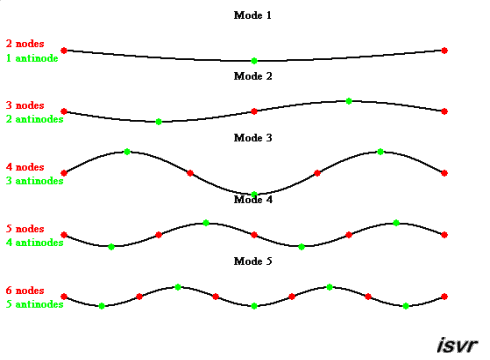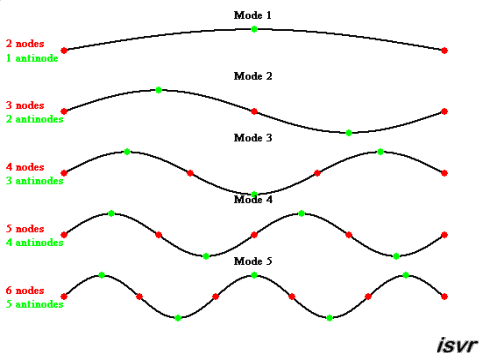
Each of these vibration
patterns is called a mode. For each of these modes, there will be
locations on the string with maximum displacement (displacement antinodes) and
locations which do not move at all (displacement nodes). For a guitar string
fixed at both ends, these modes have wavelengths related to the length of
the string, L,
where: λ = 2L, L, 2L/3,
L/2, 2L/5,...
for each of the successive modes shown in the animation below. If
n
is the order of the mode, the corresponding wavelength is then given by:
2L/n.
Using the wavelength-frequency relationship,
v = f λ ,
it can be seen that for each of these wavelengths, there is a
corresponding frequency f = v/2L,
v/L, 3v/2L, 2v/L, 5v/2L,...
Here
v
represents the speed of transverse mechanical waves on the string. These
frequencies are also called natural frequencies of vibration of the string.
When a string is excited by plucking, the resulting vibration can be
thought of as a combination of several modes of vibration.
|